
Sigmatropic rearangements
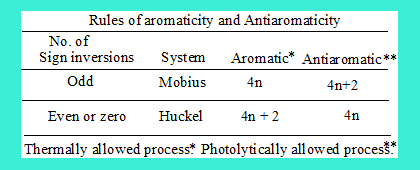
The Huckel-Mobius/Dewar-Zimmermann approach
Ms. Preeti
The analysis for a 4n system:
In the transition state if there are zero or even number of nodal planes(or phase changes)
Huckel system-anti aromatic thermally not allowed, (Mobius-aromatic) photochemically feasible.
For a 4n+2 system
In the transition state if there are zero or even number of nodal planes
Huckel system-aromatic thermally allowed (Mobius-antiaromatic). A Huckel aromatic transition state stabilises the process hence it is thermally feasible.
Note: The Orbitals chosen to decide the number of phase changes in the shift is the orbital of hydrogen and the basis set orbitals of the alkyl radical( in the case of 1,3-shift in propene it is allyl radical and in 1,5-shift the pentadienyl radical).
Here is a quote from Journal of Chemical Education (Volume 50, Number 4, April 1973)
"Thus, it can be generalised and shown that a cyclic array of orbitals with odd number of sign inversions belongs to the Mobius system, and those with zero or even number of sign inversions belongs to the Huckel system. Zimmermann applied this concept to cyclic transition states in concerted reactions. Thus a Huckel type transition state with 4n+2 electrons should be a thermally allowed (energetically favourable) process due to the aromaticity, and those with 4n electrons should be antiaromatic, and thermally disallowed. For Mobius type tranition staes, those with 4n electrons should be thermally allowed, and those with 4n + 2 electrons thermally disallowed. These rules for predicting the allowedness of a concerted pericyclic reaction are summarised in the Table. The above discussion is limited to ground state thermal reactions. For concerted photochemical reactions, the rules should be exactly opposite to those corresponding to thermal ones."
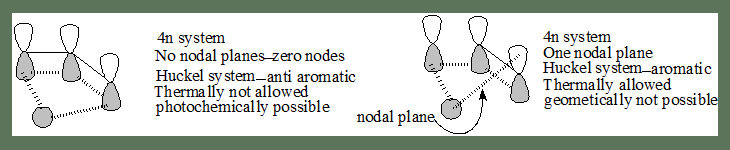

Analysis of a [3,3]-sigmatropic rearrangement, an example.
The tranistion state for this reaction is considered as due to two interacting allyl fragments
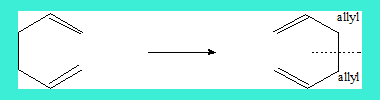

The two allyl fragments form a closed loop of π orbitals with no sign inversions in the supra-supra case and one inversion in the supra-antara case.